Research Theme 2: Steady-state experiments and coarse-grained modeling

Objective:

This Research Theme is designed as an experimental counterpart to the theoretical approach put forward in Research Theme 1. We will design and execute experiments tailored to test the validity of the coarse-grained models that act as a bridge between the pore-scale physics and the continuum scale.

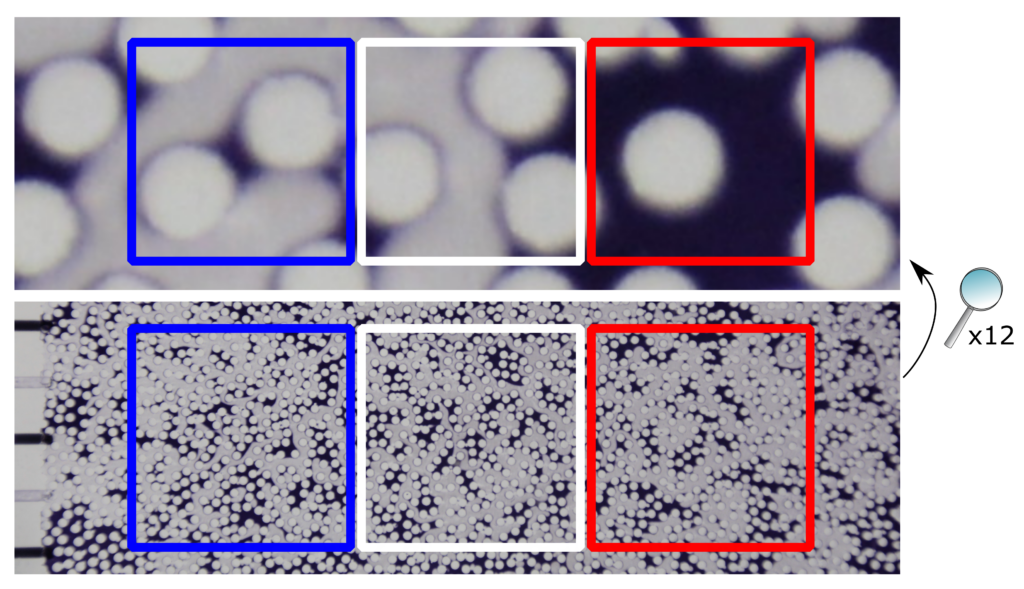
Figure: Two different levels of magnification of an experiment in which two immiscible fluids (lighter and darker colors) are simultaneously injected in a porous medium formed by a 3D printed array of circular cylinders. In the top image, the colored squares represent the typical pore scale and we see a very heterogeneous picture: the distribution of fluid phases inside the squares look very different. In the bottom image, the squares represent an intermediate scale of the experiment for which one can have well defined average quantities: the fluid configurations inside the colored squares are statistically similar.

Description:

The experimental techniques employed to visualize and understand the dynamics of fluids moving through a porous network have experienced a fast development in recent years. The advance in computer power has had an immediate impact on the field either by simply allowing for larger data sets (necessary when dealing with larger samples) or by allowing for more powerful filtering routines (more data means also more noise). In spite of these undeniable experimental advances, many fundamental questions in the field remain unanswered, particularly around the task of establishing a theoretical level of description of multiphasic flows that is both physically sound and applicable in practical settings. By physically sound, we mean that the pore-scale physics must be fully satisfied. By applicable in practical settings, we mean that one must be able to use this theory to make predictions of quantities of relevance for large-scale systems, such as phase saturations and average currents. Research Theme 1 is devoted to the task of developing such a theory, a statistical mechanics description for immiscible and incompressible two-phase flow in porous media. This new theoretical framework necessitates new experimental designs to test its predictions. We will not only need to be able to produce high-speed and high-resolution images of the flows, but we will also need to tune properties of the porous networks in a controlled manner, something that typically is not done in most of the currently employed techniques. In order to fulfill that goal we will work with artificial porous networks produced via a modern 3D printing technique called stereolithography. This technique allows for the construction of transparent networks of controlled geometrical properties with high resolution at the pore scale while still allowing for samples large enough to yield statistical relevance.

Here are some examples of typical questions the experiments in this Research Theme will target:

  1. Considering a given set of boundary conditions (fixed flow rates or pressures), fluid properties (ex. viscosities, densities and surface tension) and network properties (porosity and single-phase permeability), what is the steady-state saturation of each fluid phase?
  2. What is the characteristic signature of the fluctuations in the phase saturation inside a given REV (representative elementary volume) and how do these fluctuations relate to the experimental conditions such as average flow rates and permeability?
  3. If a steady-state configuration is perturbed (for instance by a pulse in pressure in the system), how does the system relaxes again to the steady-state?
  4. What happens when two systems, each in a steady-state configuration are put in contact with each other? One could imagine this scenario for instance with a porous medium with two sections of different permeabilities. Will a new steady-state be reached for the combined system? If so, how does this new steady-state relates to the previous ones? This situation is somewhat analogous to putting two thermal systems at different temperatures in contact with each other. As they exchange heat, a new final state with intermediate temperature is reached. But what are the analogs of temperature, heat, and Fourier’s law of heat conduction in the context of multiphasic flows?

Deliverables

A characterization of how the steady-state in immiscible two-phase flow in porous media is reached, its dependence on fluid properties, network properties and boundary conditions and its relaxation dynamics upon perturbations.

A robust experimental protocol for testing the applicability of the coarse-grained models developed in Research Theme 1, its variables and the relations between them.

Investigators

Principal Investigator Research Theme 2: Marcel Moura.

Partners: Knut Jørgen Måløy, Alex Hansen, Eirik Grude Flekkøy, Carl Fredrik Berg, Erika Eiser

Postdoctoral Fellows and Researchers: Santanu Sinha, Paula Reis, Khobaib Khobaib

PhD students: Hursanay Fyhn, Federico Lanza, Håkon Pedersen.

Selected articles:

A. Hansen, E. G. Flekkøy, S. Sinha and P. A. Slotte, “A statistical mechanics framework for immiscible and incompressible two-phase flow in porous media,” Advances in Water Resources 171, 104336 (2023).

S. Roy, H. Pedersen, S. Sinha and A. Hansen, “The Co-Moving Velocity in Immiscible Two-Phase Flow in Porous Media,” Transport in Porous Media 143, 69 (2022).

K. J. Måløy, M. Moura, A. Hansen, E. G. Flekkøy, and R. Toussaint, “Burst Dynamics, Upscaling and Dissipation of Slow Drainage in Porous Media,” Frontiers in Physics 9, 718 (2021). M. Moura, K. J. Måløy, E. G. Flekkøy, and R. Toussaint, “Intermittent dynamics of slow drainage experiments in porous media: Characterization under different boundary conditions,” Frontiers in Physics 7, 217 (2020).